How to solve the graph problem?

D

dc65k2021-02-04 07:31:29

Mathematics

dc65k, 2021-02-04 07:31:29

Hello everyone, can you please tell me how to solve the following problem?
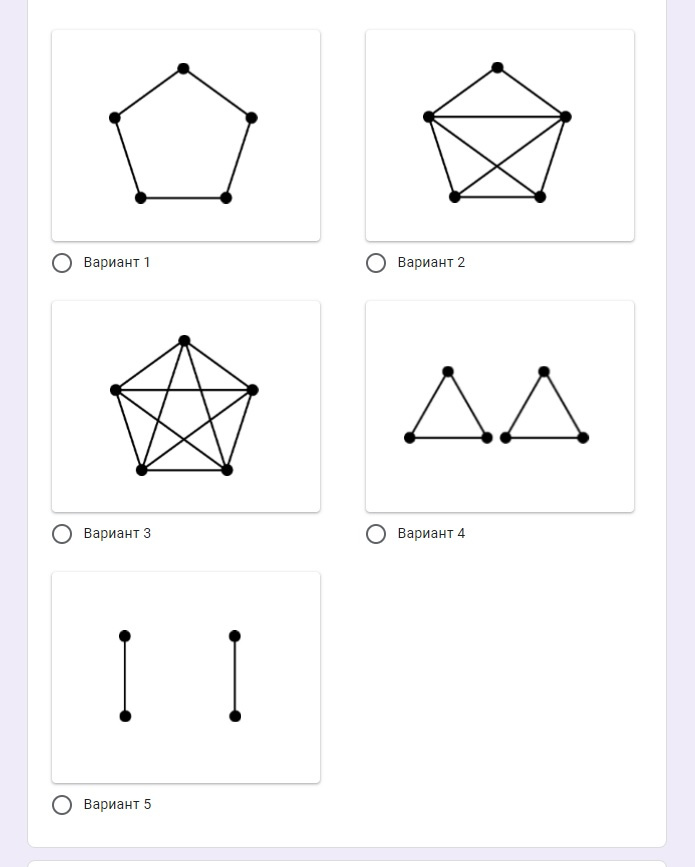
There is a statement "Every connected graph has one or more vertices of degree 2". Below are 5 graphs with 1 or more connected vertices. Which of the 5 graphs is a counterexample of the described statement?

Reply

Answer the question

In order to leave comments, you need to log in

2 answer(s)

W

Wataru, 2021-02-04
@dc65k

A counterexample is one that refutes a statement.
Statement - every connected graph has at least one vertex of degree 2.
That is, a counterexample is a connected graph that has no vertices of degree 2. The
degree of a vertex is how many edges it contains. Can you find such a graph in the picture?

U

U235U235, 2021-02-04
@U235U235

Write down the degrees of each vertex and the answer will be obvious. There is no need to even think about it.