Answer the question
In order to leave comments, you need to log in
How to fix the error in the calculations by the Runge-Kutta method (4 orders)?
Good day!
There was a problem with calculating the values for plotting the Lorentz attractor.
The fact is that already at 2 - 3 iterations, very large values of the coefficients begin to appear.
The code:
import matplotlib.pyplot as plt
from mpl_toolkits.mplot3d import Axes3D
sigma = 10
r = 28
b = 8 / 3
def LorenX(x,y):
return sigma * (x - y)
def LorenY(x,y,z):
return x * (r - z) - y
def LorenZ(x,y,z):
return x * y - b * z
def rg4(x0,y0,z0,dt = 0.0001):
x = x0
y = y0
z = z0
arrx = [0]
arry = [0]
arrz = [0]
fig = plt.figure()
ax = fig.add_subplot(111, projection='3d')
for i in range(10000):
arrx.append(x)
arry.append(y)
arrz.append(z)
k1 = LorenX(x,y)
m1 = LorenY(x,y,z)
p1 = LorenZ(x,y,z)
k2 = LorenX(x + k1/2,y + m1/2)
m2 = LorenY(x + k1/2,y + m1/2,z + p1/2)
p2 = LorenZ(x + k1/2,y + m1/2,z + p1/2)
k3 = LorenX(x + k2/2,y + m2/2)
m3 = LorenY(x + k2/2,y + m2/2,z + p2/2)
p3 = LorenZ(x + k2/2,y + m2/2,z + p2/2)
k4 = LorenX(x + k3,y + m3)
m4 = LorenY(x + k3,y + m3,z + p3)
p4 = LorenZ(x + k3,y + m3,z + p3)
x += (dt * (k1 + 2*k2 + 2*k3 + k4) / 6)
y += (dt * (m1 + 2*m2 + 2*m3 + m4) / 6)
z += (dt * (p1 + 2*p2 + 2*p3 + p4) / 6)
ax.plot(arrx, arry, arrz)
plt.show()
rg4(1,1,1)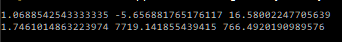
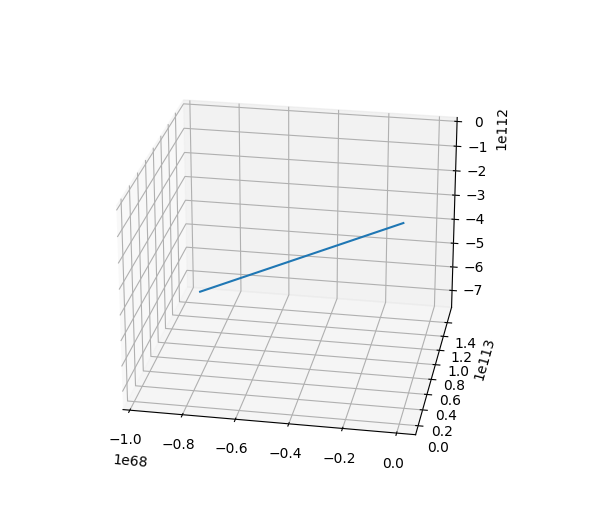
Answer the question
In order to leave comments, you need to log in
First:
# b = 8 / 3
# got integer division, b=2
b = 8.0/3
Second:
def LorenX(x,y):
# return sigma * (x - y)
return sigma * (y - x)
Third:
...from here I took the coefficients (last message with the code)Wrong copypasta. You did something strange with dt. I copied and pasted from there without any gag:
#
k1 = dt*LorenX(x,y)
m1 = dt*LorenY(x,y,z)
p1 = dt*LorenZ(x,y,z)
k2 = dt*LorenX(x + k1/2,y + m1/2)
m2 = dt*LorenY(x + k1/2,y + m1/2,z + p1/2)
p2 = dt*LorenZ(x + k1/2,y + m1/2,z + p1/2)
k3 = dt*LorenX(x + k1/2,y + m2/2)
m3 = dt*LorenY(x + k1/2,y + m2/2,z + p2/2)
p3 = dt*LorenZ(x + k1/2,y + m2/2,z + p2/2)
k4 = dt*LorenX(x + k1,y + m3)
m4 = dt*LorenY(x + k1,y + m3,z + p3)
p4 = dt*LorenZ(x + k1,y + m3,z + p3)
x += (k1 + 2*k2 + 2*k3 + k4) / 6
y += (m1 + 2*m2 + 2*m3 + m4) / 6
z += (p1 + 2*p2 + 2*p3 + p4) / 6Didn't find what you were looking for?
Ask your questionAsk a Question
731 491 924 answers to any question